Lecture 6 - Probability
72
Why should we understand
probability? Is probability
essential for physician?
Example 1: Genetic Counseling: A couple has a baby
with a genetic defect. They are considering having
another baby. What is the likelihood that the second child
will have a genetic defect also?
Example 2: Prognosis; A physician is considering several
therapies for the treatment of a patient. Which therapy
should be used? Each therapy produces a result that is
somewhere between success and failure. The final choice
is “weighed the probability” against the others.
Example 3: Is a food additive carcinogenic? An
investigator explores this in an experiment that compares
two groups. Some of the treated individuals develop
cancer and only few of the controls develop cancer.. Is the
excess number of cancers meaningful (higher probability
than control)?
Example 4: Smoking and Cancer: Lung cancer occurs
commonly in smoker but only sometimes in non smokers.
Probability of other factors related to a variable outcome.
Probabilities are a tool in decision making, and the key
to understand inferential statistics
Example 5: The data below shows the finding of a
survey. Is living near electricity transmission equipment
associated with occurrence of cancer?
Cancer Not
Near 200 1646 11%
Not 50 7289 1%
Among those living near electricity equipment, 11% have
cancer. Among those living elsewhere, only 1% have
cancer. Is this a meaningful difference?
The difference (if significant) in this example is
reflected for population and called inference
Probability is the bridge between Descriptive Statistics
and Inferential Statistics
The sample space is the universe, or collection, of all
possible outcomes.
The probability (P) of the occurrence of event (E) is equal
to the number of times (E) occur (M), divided by the
number of times E can occur (N). P (E) = M\N
The concept of probability is frequently encountered in
every day communication of health workers, we may here
the physician say that a patient has 50-50 chance of
surviving, or a patient 95% has the disease.
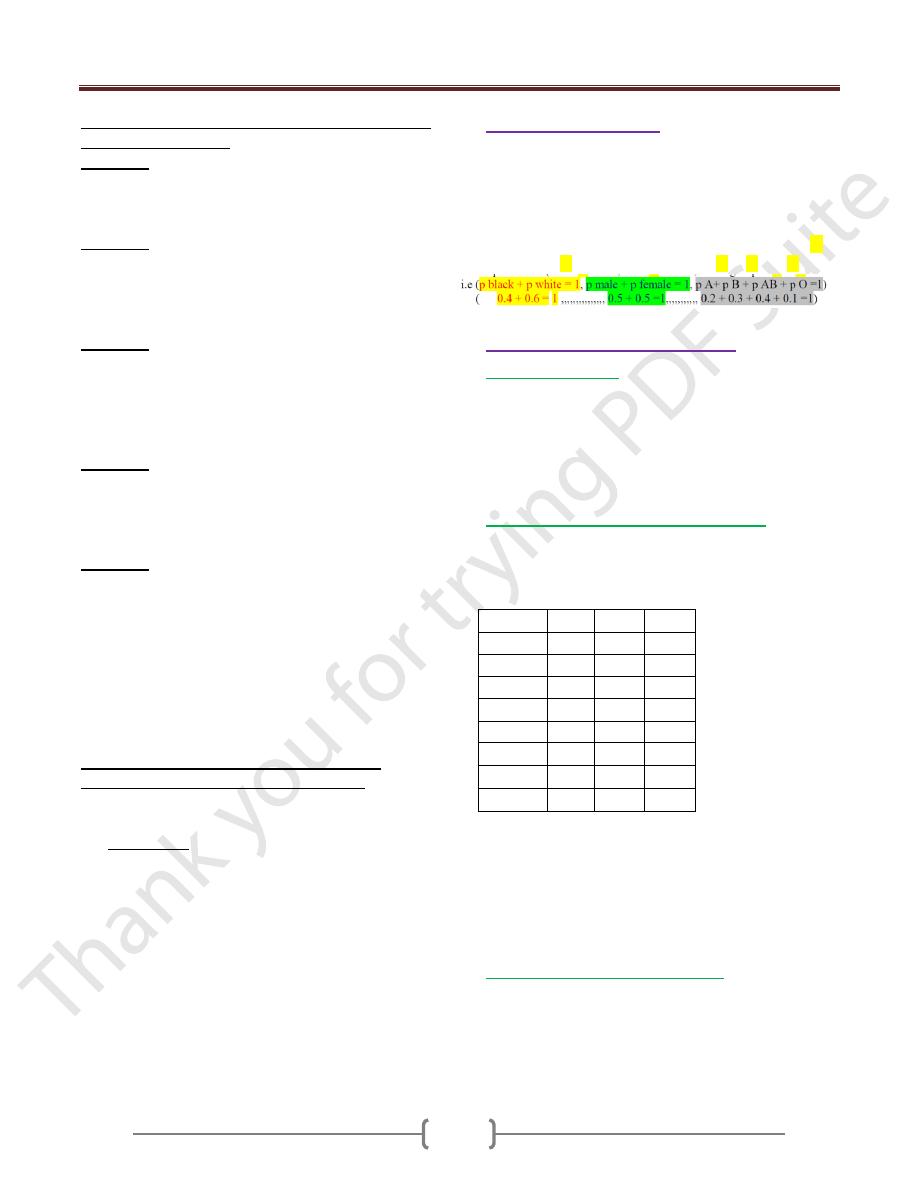
Element of probability
1) Total probability value must be between 1 & zero (0 ≤ P≤
1), no negative value.
P = 0 → Not occur. P = 1→ should occur.
P = 0.5→ 50% will occur & 50% will not occur.
2) The sum of the probabilities of mutually exclusive (can't
occur simultaneously) outcome is equal to one.(black
or
white, male
or
female, blood group A
or
B
or
AB
or
O)
There are 3 types of probability:
1) Classical Probability
:
Assume that all outcomes in the sample space are equally
likely to occur. One does not actually have to perform the
experiment to determine the probability.
Ex: When a single die is rolled, each outcome has the
same probability of occurring Since there are 6 outcomes,
each outcome has a probability of 1/6
2) Empirical Probability (Relative frequency):
Depend on actual experience to determine the likelihood
of outcomes
Ex: In a study, we have the following table for serum
cholesterol of 1047 male patients aged 40-59 year.
S.choles.
F
R.F
C.R.F
<160
31
3
3
160-199
134
12.8
15.8
200-239
358
34.2
50
240-279
326
31.1
81.1
280-319
145
13.7
94.8
320-359
43
4.1
98.9
360 +
12
1.2
100
totals
1047
100%
----
The probability to get individuals with serum cholesterol
of 820-319 is 145 / 1047 = 13.7%
Probability to get those below 200 is (31 +134)/ 1047 =
15.8
So we can express probability in terms of relative
frequency or cumulative relative frequency.
3) Subjective (personalistic) Probability
:
Based on person’s experience and evaluation of the situation
But does not rely on the repeatability of any process
Lecture 6 - Probability
73
A physician might say that on the basis of his diagnosis,
there is a 30% chance that the patient will need an
operation.
If a doctor says “you have a 50% chance of recovery,”
the doctor believes that half of similar cases will recover
in the long run.
Presumably, this is based on knowledge, and not on a
whim. The benefit of stating subjective probabilities is
that they can be tested and modified according to
experience.
Joint probability
:
It is the probability that the events (2 or more, E
1
, E
2
..etc)
can occur simultaneously. We have the following 2 rules:
1) Multiplication rule (And, ∩, both).
a) Independent events (E
1
not affected by E
2
).
P (E
1
∩E
2
) = P (E
1
) x P (E
2
)
Two events are statistically independent if the chances, or
likelihood, of one event is in no way related to the
likelihood of the other event. Individual is male with red
hair.
EX: Event A = “a woman is hypertensive”
Event B = “her husband (not relative) is hypertensive”.
The assumption of independence seems reasonable since
the two persons are not genetically related. If the
probability of being hypertensive is 0.07 for woman and
0.09 for man, then the probability that BOTH the woman
and her husband are hypertensive is:
P(A and B) = P(A) x P(B) = 0.07 x 0.09 = 0.0063
Ex: The probability that an individual belonging to blood
group A is 0.42, and the individual being a football player
is 0.50. What is the probability of the individual both
belonging to blood group A & being football player?
Since the events are independent → P (E
1
∩E
2
) = P (E
1
) x
P (E
2
) = 0.42 x 0.50 = 0.21
b) Dependent events (E
1
affected by E
2
).
P (E
1
∩E
2
) = P (E
2
) x P (E
1
/ E
2
)
EX: Probability of being male 1s 0.5, and that that male
being bold is 0,05. What is the probability of both being
male and bold?
Since the events are dependent→ P (E
1
∩E
2
) = P (E
2
) x P
(E
1
/ E
2
) =0.5 x 0.05/0.5= 0.005
EX: the chance that person has Huntington ’s chorea is
0.0002 (if the parent does not have Huntington’s Chorea).
An offspring of a person with Huntington’s Chorea has a
50% chance of contracting Huntington’s Chorea
(offspring with chorea giving that his father had chorea).
Probability 2 persons have Huntington’s Chorea =
P(A and B) = 0.0002 X 0.0002= 0.00000008
Probability both parent and child have Huntington’s
Chorea = P(A) P(B|A) = 0.0002 x 0.5 = 0.0001
*Conditional probability: Probability of an event
occurring (E
1
) giving that the other event (E
2
) has already
occur.
P (E
1
/ E
2
) =P (E
1
& E
2
)/ P E
2
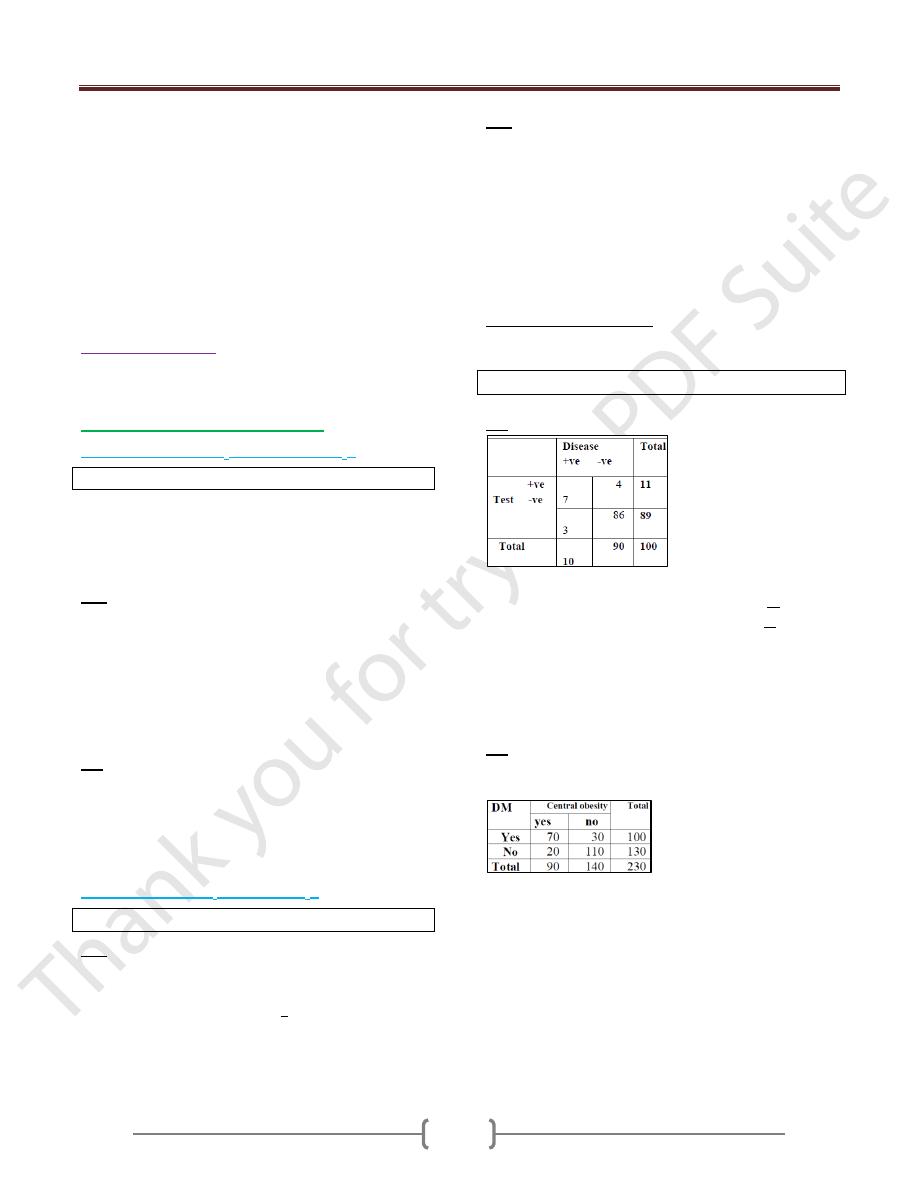
Ex: Using the information of table below:
Calculate:
1- The probability of selection person dis. +ve & test +ve.
2- The probability of selection person dis. -ve & test -ve.
As the variables are dependent, so P (E
1
∩E
2
) = P (E
2
)
xP (E
1
/ E
2
)
1- P (dis. +ve & test +ve.) = 11/100 x 7/11 = 7/100
2- P (dis. -ve & test -ve.) = 90/100 x 86/90 = 86/100
Ex: Probability of DM patient given that he has central
obesity=70/90, the denominator only patient with Central
obesity.
P(A/B)=P(A+B)/B
Lecture 6 - Probability
74
2) Additional rule. (Or, U, either)
a) Mutually exclusive events (can't occur together).
Two events are mutually exclusive if they cannot occur
at the same time.
P (E
1
U E
2
) = P (E
1
) +P (E
2
)
EX: if a baby has a 0·04% chance of being homozygous for the sickle
cell gene and a 3·92% chance of being a heterozygote, then the
probability that it carries the gene either as a homozygote or as a
heterozygote is 0·04 + 3·92 = 3·96%.
Ex: The probability that an individual belonging to blood
group A is 0.4 and the individual belonging to blood
group B is 0.3. What is the probability of the individual
belonging to blood group A or B?
As the variables are mutually exclusive events (can't
occur together), so:
P (E
1
UE
2
) = P (E
1
) +P (E
2
) = 0.4 +0.3 = 0.7
a) Not mutually exclusive events (can occur together).
P (E
1
UE
2
) = P(E
1
) +P(E
2
) )-P(E
1
and E
2
)
Ex: Using the information of table above, calculate the
probability of selection person dis. -ve or test -ve.
As the variables are not mutually exclusive events (can
occur together), so:
P (E
1
UE
2
) = P (E
1
) +P (E
2
)-P (E
1
and E
2
)
= [(90/100) + (89/100)] - (86/100) = 0.93
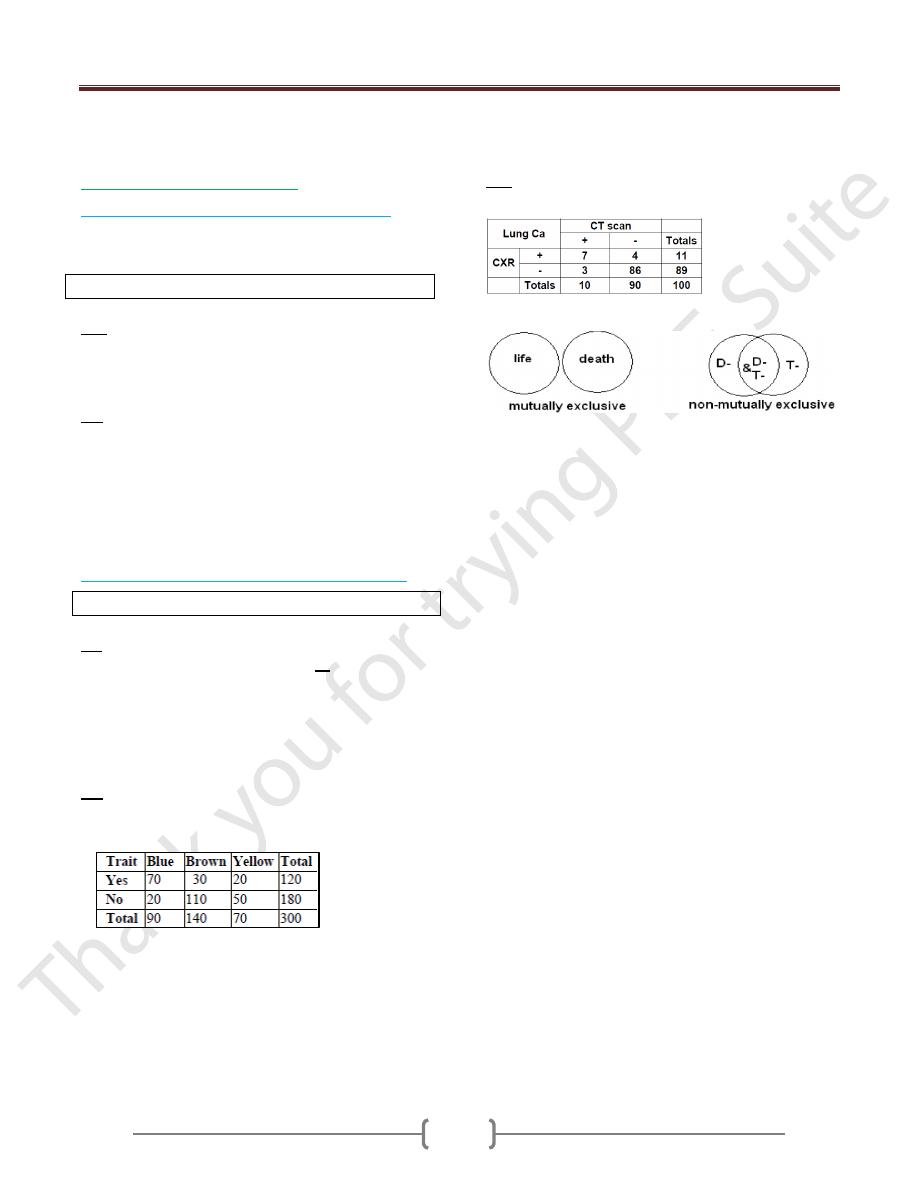
Ex: Suppose a certain ophthalmological trait determines
the eye color, 300 randomly selected individuals are
studied and the results are as follow:
1- P (trait) = 120/ 300 = 0.4
2- P (No trait) = 180/300 = 0.6
3- P (Blue eye) = 90/300 = 0.3
4- P (Brown eye)= 140/300 = 0.46
5- P (Yellow eye) = 70/300 = 0.24
6- P (Blue or Brown eye) = (90/300) + (140/300) =0.76
7- P (Blue eye and trait) = (120/300) x (70/120) = 0.23
8- P (Blue eye or trait) = (90/300) + (120/300) -(70/300)
= 0.23
9- P (Brown eye / trait) = (30/120) = 0.25
EX: From table below, what is the probability to have a
person that is CT scan –ve or CXR negative?
= 0.90 + .089 - 0.86 = .93 = 93%
The ideas of mutually exclusive and independence are
different. The way we work with them are also different.
We say the events of “heads” and “tails” in the outcome
of a single coin toss are mutually exclusive. A coin toss
cannot produce heads and tails simultaneously. Weight of
an individual classified as “underweight”, “normal”,
“overweight” The outcomes on the first and second coin
tosses are statistically independent and, therefore,
probability [“heads” on 1st and “tails” on 2nd ] =
(1/2)(1/2) = 1/4.
The distinction between “mutually exclusive” and
“statistical independence” can be appreciated by
incorporating an element of time. “heads on 1st coin toss”
and “tails on 1st coin toss” are mutually exclusive.
Probability [“heads on 1st” and “tails on 1st” ] = 0
Statistical Independence “heads on 1st coin toss” and
“tails on 2nd coin toss” are statistically independent
Probability [“heads on 1st” and “tails on 2nd ” ] = (1/2)
(1/2)
Group Work: Table below summarizes results of a study
to evaluate the CXR as diagnostic test for TB. The study
involved 240 patients with symptoms of fever and
prolong cough who were seen at a medical facility for the
diagnosis and treatment of chest diseases. Sputum
specimens obtained from each of the patients and
examined and the results obtained from CXR and sputum
exam were cross tabulated.

Lecture 6 - Probability
75
a. What is the probability that a patient has sputum +ve?
183/240= 76.25%
b. What is the probability that a patient has sputum -ve?
57/240=
c. What is the probability that a patient has CXR +ve?
184/240
d. What is the probability that a patient has CXR -ve?
56/240=
e. What is the probability that a patient has a positive CXR
and +ve sputum?
175/240 = 0.7292 OR = (183/240) (175/183)
f. What is the probability that a patient has CXR –ve and
sputum -ve?
48/240 = .20 OR = (57/240) (48/57)
g. What is the probability that a patient has CXR +ve giving
that sputum +ve?
175/183 = .9563 OR = (175/240) / (183/240) = 175/183
h. What is the probability that a patient with sputum -ve has
a negative CXR?
48/57 = .8421 OR = (48/240) / (57/240) = 48/57
i. What is the probability that a patient with sputum -ve has
a positive CXR?
9/57 = .1579 OR = (9/240) / ( 57/240) =9/57
j. What is the probability that a man with sputum +ve has a
negative CXR?
8/183 = .0437 OR = ( 8/240)/(183/240) = 8/183
k. What is the probability that a man with a positive CXR
has sputum +ve?
175/184 = .9511 OR = ( 175/240)/(184/240) = 175/184
l. A clinic similar to the one that conducted the CXR study
examines a man with symptoms of cough and prolong
fever and decides to order the CXR, which comes back
negative. What is the likelihood that this patient has TB?
What is the likelihood that he does not have it?
The probability that TB patient gives -ve CXR = 8/56 =
.1429
The probability that he does not have TB is = 48/56 =
.8571
m. Based on data in the above table, what is the probability
that a man who visits the clinic has a positive CXR or
sputum, or P(T+ or D+)?
= (148/240)+(183/240)-(175/240) = 192/240 = .80
n. Consider the probabilities P(Sputum+/CXR+) &
P(CXR+/Sputum+). Which would be more informative to
a physician who is interested in establishing a diagnosis
of TB in a patient?
Physicians frequently must interpret positive or negative
test results in an attempt to judge the likelihood of disease
in a given patient. Therefore, the probability of most
interest (from a diagnostic point of view) is the
probability of disease given a positive test result,
P(Sputum+/CXR+) or P(D+/T+).
EX: Cystic fibrosis is an autosomal recessive disease, and
it is manifested when a person carries two mutant alleles.
Parents of affected children are heterozygous carriers. A
healthy 26-year-old male comes for a routine health
maintenance examination. He recently got married, and
he is currently planning to have children. His younger
brother was recently diagnosed with cystic fibrosis, and
he wants to know his chances of carrying the abnormal
allele. Which of the following is the best response?
A) He has 25% chance of being a carrier
B) He has 50% chance of being a carrier T
C) He has 75% chance of being a carrier
D) He has 100% chance of being a carrier
The chance of being a carrier (i.e., the chance of carrying
one mutant allele) is 1/2, the chance of having the disease
(i.e., the chance of having two mutant alleles) is ¼, and
the chance of having both normal alleles is ¼.
